DYNAMIC ANALYSIS OF THE MATHEMATICAL MODEL OF THE SPREAD OF CHOLERA WITH VACCINATION STRATEGIES
Authors: Nur Safitri Abdul, Lailany Yahya, R Resmawan, Agusyarif Rezka Nuha
This research discusses the math model of spreading cholera disease with a mathematical strategy of math model constructed by considering a vaccination strategy. In addition, there is a population of hyper infectious and less infectious bacteria so the model of SVIR-BhiBli type, by. The model is formed in the form of determination of fixed point, determination of basic reproductions numbers, analyzing the equilibrium point and sensitivity analysis. The equilibrium analysis produces two equilibrium points of a immediate-free equilibrium point of aceletotic local if and endemic equilibrium points will be stable local asymptotics if . Furthermore, numerical simulation that the increase in vaccination rate influences on the decline in value while increased rate of vaccine depreciation can increase the value of . In addition, sensitivity analysis shows that if the parameter is enhanced while other contrast parameters will contribute to the increase in value, as a result can increase the rate of transmission of cholera disease. Whereas if the parameter is enhanced while other contrast parameters will contribute to the decrease in value, as a result of the dissemination of the disease can be pressed very significantly.
Published in Barekeng (SINTA 2)
GOODWIN MODEL WITH CLUSTERING WORKERS' SKILLS IN INDONESIAN ECONOMIC CYCLE
Authors: Sri Lestari Mahmud, Resmawan Resmawan, Sumarno Ismail, Nurwan Nurwan, Febriani Taki
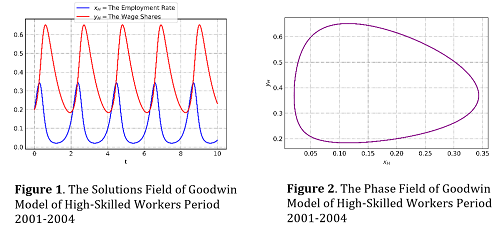
The economic model which deals with the economic cycle is Goodwin's Model. It presents the relationship between the employment rate and wage shares. In this study, the modification model was made, taking into three types of workers, namely high, medium, and low-skilled workers. Studies of the model are conducted by determining the equilibrium point and its stability analysis. Furthermore, a numerical simulation is given to see which model satisfies the ideal of Goodwin‘s model cycle prediction by using Indonesian data from 2000 to 2020. In the end, an investigation into the effects of reducing the wage gap between the three types of workers was conducted. The results showed two equilibrium points, namely The Equilibrium Point without Employment Rate and The Wages Share (T1) and the Existence Equilibrium Point of Employment Rate and Wages Share (T2). T1 achieves a stable node condition when ScQ<d+pi+et while T2 reaches a stable center condition when ScQ>d+pi+et. The simulation showed Goodwin's model of high- and low-skilled workers produced the ideal of Goodwin model cycle predictions, whereas Goodwin's model of medium-skilled workers and the entire economy (capitalist) didn’t produce the ideal of Goodwin model cycle predictions. Eventually, the effects of reducing the wage gap make the economy unstable.
Published in Cauchy (SINTA 2)
A discrete-time fractional-order Rosenzweig-Macarthur predator-prey model involving prey refuge
Author: Hasan S. Panigoro, Emli Rahmi, Novianita Achmad, Sri Lestari Mahmud, R. Resmawan, Agusyarif Rezka Nuha
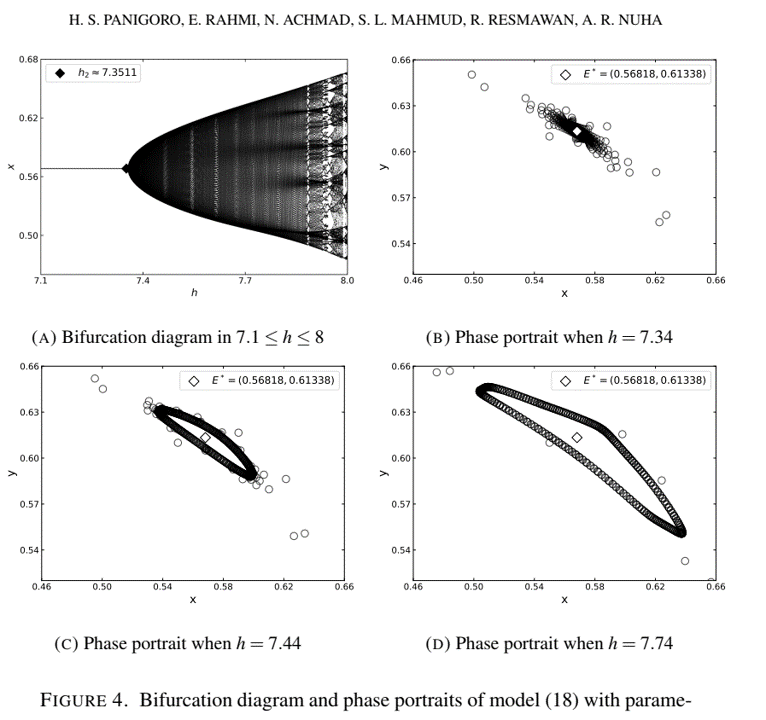
In this article, the dynamical behaviors of a discrete-time fractional-order Rosenzweig-MacArthur model with prey refuge are studied. The piecewise constant arguments scheme is applied to obtain the discrete-time model. All possible fixed points and their existence conditions are investigated as well as the local behavior of nearby solutions in various contingencies. Numerical simulations such as the time series, phase portraits, and bifurcation diagrams are portrayed. Three types of bifurcations are shown numerically namely the forward, the period-doubling, and Neimark-Sacker bifurcations. Some phase portraits are depicted to justify the occurrence of those bifurcations.
Published in Communications in Mathematical Biology and Neuroscience (Q3)
Analysis of The Rosenzweig-MacArthur Predator-Prey Model with Anti-Predator Behavior
Author: Ismail Djakaria, Muhammad Bachtiar Gaib, Resmawan Resmawan
This paper discusses the analysis of the Rosenzweig-MacArthur predator-prey model with anti-predator behavior. The analysis is started by determining the equilibrium points, existence, and conditions of the stability. Identifying the type of Hopf bifurcation by using the divergence criterion. It has shown that the model has three equilibrium points, i.e., the extinction of population equilibrium point (E0), the non-predatory equilibrium point (E1), and the co-existence equilibrium point (E2). The existence and stability of each equilibrium point can be shown by satisfying several conditions of parameters. The divergence criterion indicates the existence of the supercritical Hopf-bifurcation around the equilibrium point E2. Finally, our model's dynamics population is confirmed by our numerical simulations by using the 4th-order Runge-Kutta methods.
Sensitivity Analysis of Mathematical Model of Coronavirus Disease (COVID-19) Transmission
Coronavirus Disease (henceforth, COVID-19) has shocked many thanks to its very rapid spread. Firstly, identified to occur in Wuhan city of China, the disease has shortly become one of the main talking points as it reached the whole world and took thousands of death tolls in a very short period. The disease somewhat instigates all parties to conduct active measures in finding options to the best treatments and anticipatory means to prevent damage on a much wider scale. From a mathematical perspective, the concern is closely related to the implementation of mathematical models to identify potential solutions.
Mathematical modeling is one of the key tools in epidemic preparation, including the COVID-19 pandemic. The system allows one to comprehend and identify the correlation between COVID-19 spread and several epidemiology parameters, conduct preparatory measures for future planning, and implement best practices of pandemic treatment. Previous studies, albeit little in number, have begun to address this problem and design mathematical model for COVID-19 transmission [1][2][3][4]. The model involved accurate and effective public health interventions. On top of that, a study comparedbetween the outbreaks of current COVID-19 and previous MERS disease that spread in Middle Eastern countries and Korea [5]. Other studies designed mathematical models that predict COVID-19 cases in different countries [6][7].
Several models proposed in previous studies have discussed that the virus started from an unknown source and eventually began to spread to the human population. The virus source, further referred to as reservoir variable, is suspected to be the place of first infection-to-human case. The present study introduces a different approach to mathematical modeling to the virus transmission by also involving the epidemiology parameters; a variable that is not discussed in previous studies. Previous models have assumed that the virus transmission only occurs in interactions between individuals that have contracted the virus; differing from that, this article takes into consideration transmission cases caused by susceptible individuals and exposed individuals. It views the importance of involving such parameters, considering the number of infections that occurred in the interaction between exposed individuals yet to be detected as infected. Moreover, the model lays its emphasis on the pattern of transmission between humans after the virus has become epidemic or pandemic, therefore, disregarding the reservoir variable. The model thus overlooks the process of first human infection and focuses on how the virus has spread within human-to-human interactions. In addition to that, the model employs new parameters representing death cases of COVID-19, pertaining to the fact that the virus has taken numbers of the death tolls. The model also takes into account cases of quarantined individuals that were identified to be exposed to the virus.
The following section elaborates on the construction of mathematical models in this study. Further, the article presents the research results in the form of model analysis. Within this section, the study focuses on the construction of basic reproductive number and sensitivity analysis to identify which parameter is the most sensitive to the change in basic reproductive number value. Finally, the last section proposes several conclusions to the research findings and discussion.
Kategori
Arsip
- July 2023 (3)
- January 2023 (1)
- December 2022 (1)
- August 2022 (1)
- July 2022 (6)
- March 2022 (1)
- September 2021 (2)
- October 2020 (1)
- July 2020 (2)
- April 2020 (1)
- November 2019 (1)
- September 2019 (1)
- August 2019 (2)
- July 2019 (1)
- May 2019 (1)
- February 2019 (1)
- September 2018 (2)
- August 2018 (1)
- July 2018 (4)
- June 2018 (5)
- September 2017 (2)
- August 2017 (1)
- April 2017 (1)
- October 2016 (1)
- September 2016 (2)
- September 2015 (4)
- June 2015 (1)
Blogroll
- 01 Sistem Informasi Akademik
- 02 Repository UNG
- 03 Universitas Negeri Gorontalo
- 04 Beasiswa DIKTI
- 05 Beasiswa LPDP
- 06 BookFi
- 07 Indonesian Mathematical Society
- 08 EBSCOhost
- 09 Library Genesis
- 10 Khan Academy
- 11 Blog Pribadi
- 12 Twitter
- 13 Facebook
- 14 Pdf Drive
- 15 Pangkalan Data UNG
- 16 Differential Equation
- 17 Math is Fun
- 18 Jambura Journal of Mathematics
- 19 OSF
- 20 Sci-Hub
- 21 Researchsquare
- 22 Kalkulator Math
- 23 Gometa
- 24 Microsite
- 25 Wordwall
- 26 Science Direct
- 27 BSRE BSSN
- 28 OpenAI
- 29 Quillbot
- 30 Perplexity
- 31 Citation FInder
